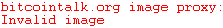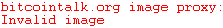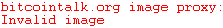It is often possible to see the Chicago skyline from sea-level 60 miles away across Lake Michigan. In 2015 after photographer Joshua Nowicki photographed this phenomenon several news channels quickly claimed his picture to be a “superior mirage,” an atmospheric anomaly caused by temperature inversion. While these certainly do occur, the skyline in question was facing right-side up and clearly seen unlike a hazy illusory mirage, and on a ball-Earth 25,000 miles in circumference should be 2,400 feet below the horizon.
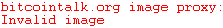
From Genoa, Italy 70 feet above sea-level, the island of Capraia 102 miles away can often be seen as well. If Earth were a ball 25,000 miles in circumference, Capraia should always remain hidden behind 5,605 feet, over a mile of supposed curvature.
Also from Genoa, on bright clear days, the island of Elba can be seen an incredible 125 miles away! If Earth were a ball 25,000 miles in circumference, Elba should be forever invisible behind 8770 feet of curvature.
In Chambers’ Journal, February 1895, a sailor near Mauritius in the Indian Ocean reported having seen a vessel which turned out to be an incredible 200 miles away! The incident caused much heated debate in nautical circles at the time, gaining further confirmation in Aden, Yemen where another witness reported seeing a missing Bombay steamer from 200 miles away. He correctly stated the precise appearance, location and direction of the steamer all later corroborated and confirmed correct by those onboard. Such sightings are absolutely inexplicable if the Earth were actually a ball 25,000 miles around, as ships 200 miles distant would have to fall approximately 5 miles below line of sight!
Of course the buildings of the Chicago skyline are right-side up. You're talking about less than a single degree of angular difference over a 59 mile stretch. How big of a perceptual difference do you think that is going to make? That's less than the same amount of differential change that you would notice in the sun's position over a duration of 4 minutes.
Of course you can see the Chicago skyline from 59 miles away. Notice what you don't see in the picture? The ground, nor the bottoms of the buildings. Why? Because curvature.
Distance to horizon = sqrt(2*r*h)
Radius of earth in meters = ~6,378,100m
h = height in meters
Let's say you have a 500 ft tall building, i.e. h=~152.4m. From the top of that building to the horizon, you get sqrt(2*6,378,100*91.44) = ~44.122km. Okay, so, little over 44km to the horizon.
Now, why can you see the bulk of a 500 foot tall building at sea level if its 60 miles (i.e. ~96.56km) away?
Simple: atmospheric refraction.
At sea-level, atmospheric refraction bends light at about 0.5 degrees, which is slightly larger than the entire diameter of the sun as it appears in the sky. From 60 miles away, the Chicago skyline, would occupy far less than 0.5 degrees of your perceptual scope. In fact, a 500 foot building would only occupy ~0.08 degrees of perceptual scope at a distance of 60 miles.
What does this mean? It means that you could simply compensate for atmospheric refraction by imagining that 500 foot building to be more than 6 times its original height, or at minimum 3000 ft, or ~914.4m
So, what's the distance to the horizon from a vantage point of 914.4m? Sqrt(2*6,378,100*914.4) = ~108.077km, or
67.155mi.Now, subtract the actual distance of 60mi from this, and you get
7.155 mi. This is the distance you then use to determine how much of the building you would expect to see blocked by curvature. It's a little over 32 feet. In other words, you would still see over 460 feet of that 500 foot building from 60 miles away at sea-level. This is entirely consistent with that photo of the skyline, where the ground is not visible, but the bulk of the buildings are.
This applies to all of your cute little images. Jesus Christ, research this!